This article throws light upon the top five applications of time value techniques. The applications are: 1. Sinking Fund Problems 2. Capital Recovery Problems 3. Compound Growth Rate Problems 4. Interest Rate Problems 5. Valuation Problems.
Time Value Techniques: Application # 1.
Sinking Fund Problems:
A financial manager may have to determine the amount of annual payments so as to accumulate a specified sum of money on a future date to redeem an existing liability or provide funds for replacement of an existing asset.
He can easily do so by making use of compound value technique:
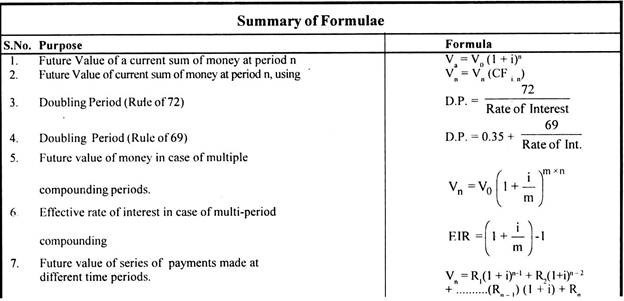
Vn =(R)(ACFi, n)
or, R = Vn/ACFin
Illustration 1:
A company has Rs. 2,00,000 6% Debentures outstanding today. The company has to redeem the debentures after 5 years and establishes a Sinking Fund to provide funds for redemption. Sinking Fund Investments earn interest @ 10% p.a. The investments are made at the end of each year. What annual payment must the firm make to ensure that the needed Rs. 2,00,000 is available on the designated date?
Solution:
R = Vn/ACFin
Referring to the Annuity Compound Factor Tables, we find that at (i) 10 percent and n = 5 years, the compound value of an annuity of Re. 1, is 6.105.
... R = 2,00,000/6,105
= Rs. 32,760.03
Hence, the firm should make annual payment of Rs. 32,760.03 for 5 years.
Time Value Techniques: Application # 2.
Capital Recovery Problems:
A financial manager may also be interested to know the amount of equal installment to be paid every year to discharge a specified amount of loan raised from some financial institution at a given rate of interest and in a fixed period.
It can be easily calculated by applying the present value techniques as below:
Vn =(R)(ADFi, n)
or, R = V0/ADFi, n
Illustration 2:
A company has raised a loan of Rs. 5,00,000 from a financial institution at 8% p.a. rate of interest. The amount has to be paid back in 5 equal annual installments. What shall be the size of installment?
Solution:
R = V0/ADFi, n z
Referring to the Annuity Discount Factor Tables at (i) 8 percent and n, 5 years, we get 3.993
R = 5,00,000/3,993
= Rs. 1,25,219.13
So, the firm should pay an annual installment of Rs. 1,25,219.13 for 5 years.
Time Value Techniques: Application # 3.
Compound Growth Rate Problems:
Sometimes a finance manager may have to calculate the compound rate of growth over a period of time, e.g.; for sales or profits. He can easily calculate such compound rate of growth by making use of Compound Factor Tables as demonstrated in the following illustration.
Illustration 3:
From the following data available for ABC Co. Ltd.; you are required to calculate compound rate of growth in profits.
Solution:
Vn =V0 (ACFi, n)
where CFi, n is compound factor at (i) percent and (n) period.
or, 100 = 60(CFi, n)
or CFi, n = 100/60 = 1.666
Using Compound Factor Tables for 6 years row we find that the nearest value to 1.666 is 1.67 at 9%, Hence the compound rate of growth is nearly 9%.
Time Value Techniques: Application # 4.
Interest Rate Problems:
The time value techniques of compounding and present value can also be applied to calculate the implicit rate of interest in certain situations, as illustrated below.
Illustration 4:
An investment company offers to pay Rs. 20,304 at the end of 10 years to investors who deposit annually Rs. 1000. What interest rate is implicit in the offer?
Solution:
Future Value of an Annuity V = V0 (ACFi, n)
where, R = Annual Payment
ACFi, n = Annuity Compound Factor at (i) rate of interest and (n) period.
Substituting the values,
20,304 = 1000 (ACFi, n = 10years)
or ACFin = 20,304/1,000 = 20.304
Using Annuity Compound Factor Tables, we find that when n = 10 years and ACF = 20.304, the rate of interest is 15%
Hence the rate of interest implied in the offer is 15%.
Illustration 5:
A company offers to pay you Rs. 4007 annually for 5 years if you deposit Rs. 16,000 initially with the company. What interest rate do you earn on the deposit?
Solution:
Using Present Value Annuity Tables
V0 =(R)(ADFi, n)
or, 16000 = 4007 (ADFi, n)
or ADFi, n = 16000/4007 = 3.993
From the Annuity Discount Tables, we find that for annuity discount factor 3.993 for a period of 5 years, the rate of interest is 8%
Hence, the rate of interest earned on deposit is 8% p.a.
Time Value Techniques: Application # 5.
Valuation Problems:
The techniques of time value of money are also applied in dealing with the valuation problems of bonds, debentures, etc.