For listed options the Black-Scholes model can be directly applied for valuation and it is commonly used by practitioners for such a purpose. However, for options that are not explicitly stated but embedded as part of the condition of another instrument and thus implicit, a less direct method (incorporating the standard binomial model) is commonly used by practitioners.
This mode of option valuation is used with respect to valuing the call provision for a bond. For purposes of assessing embedded options, it is usual to view the option bearing bond as a package of cash flows and a package of options on those cash flows.
As an example, a callable bond or one that allows an issuer to redeem the bond at a specified price prior to maturity can be viewed as a package of cash flows (coupons and principal payments) and a package of call options on those cash flows. As such the position of an investor in a callable bond can be viewed as.
Long a Callable Bond = Long an option free bond + Short a call option on the bond
Developing a value for an embedded option entails a valuation of both the bond with an embedded option and a comparable bond without an option. The difference between these two becomes the estimated value of the embedded option.
Value of Call Option = Value of option free bond – Value of callable bond
To generate comparative values for option free and option embedded bonds, the generalized procedure is to:
(1) Project cash flows for each bond, and
(2) Discount these back to present value at an appropriate interest rate.
For discounting these cash flows, standard practice is to use a risk-free rate as estimated from the term structure of interest rates. Typically, the current term structure is used as the market’s forecast of where future rates will go.
Valuation of Callable Bond:
However, for a bond with an embedded option we also need to consider the like volatility of interest rates over the maturity of the bond because the cash flows to a bond with an embedded option will depend on the path that bond prices follow over the life of the bond and because the differing bond prices will depend on the volatility of interest rates.
To properly value a bond with a call provision, we need to consider the differing interest rate scenarios that might transpire over the period to maturity of the bond.
Furthermore, we can derive the implied price of the bond given the interest rate event that occurs by means of this model. Obviously this process requires considerable programming and computer power, especially for bonds with long maturities.
Techniques for Estimating Variability of Stock Price:
There are several techniques for estimating the variability of the stock price, but the following are the two important techniques that are used in measuring the volatility of stock.
I. The first technique uses historically derived values of the standard deviation of price changes of their stock as an estimate of the standard deviation to be generated in the future. The time period of the measurements becomes important in this regard; too long a period may result in the inclusion of irrelevant observations and too short a period may exclude valuable information and not be representative. Some recommend the most recent six months’ or years’ trading data as the best measurement interval with the use of historical data.
II. An alternative way to estimate the variability of a stock is the option valuation formula. Rather than using the formula to assess the proper price of an option, we can observe the current price of an option and deduce the standard deviation of the stock price as implied in the formula.
Calculating and averaging the implied deviation over a series of past periods may provide a more accurate assessment of the expected variability of the stock than a straight forward averaging of past values. In either case, it may be necessary to adjust these derived values for possible future changes in the variability of the stock price.
Problem 1:
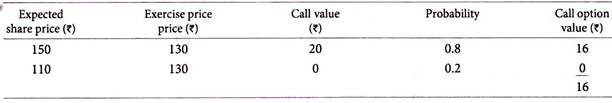
KPS Ltd.’s share price at present is Rs.120. After 6 months, the price will be either Rs.150 with a probability of 0.8 or Rs.110 with a probability of 0.2. A European call option exists with an exercise price of Rs.130. Calculate the expected value of the call option at maturity date.
Solution:
Expected value of Call option
Problem 2:
The stock of ABC Ltd. sells for Rs.240. The present value of exercise price and the value of call option are Rs.217.40 and Rs.39.60 respectively. What is the value of put option?
Solution:
Value of put option = Value of call option + P.V. of exercise price – Stock price
= Rs.39.60 + RS.217.40 – RS.240 = RS.17.00
Problem 3:
The equity shares of MNC Ltd. are selling at Rs.240 each. At the end of the holding period the share is expected to be worth any one of the following values:
Calculate the worth of European call option with exercise price of Rs.240 will (ignoring time value of money).
Solution:
Vo = (0.3 x 0) + 0.4(Rs.250 – Rs.240) + 0.3(Rs.280 – Rs.240) = Rs.16
Problem 4:
In June 30, 2016, a six-month call on Royal Ltd.’s stock with an exercise price of Rs.50 sold for Rs.4. The stock price was Rs.40. The risk-free rate was 5% per annum. How much would you be willing to pay for a put on Royal Ltd.’s stock with the same maturity and exercise price? (Given: e-rt = e-0.05×0.5 = 0.9753).
Solution:
Based on put call parity theory
P = C + PV of EP – S = Rs.4 + Rs.50 x 0.9753 – Rs.40 = Rs.4 + Rs.48.76 – Rs.40 = Rs.12.76
Problem 5:
The shares of Nabani Ltd. are trading at Rs.370.
If put options with a strike price of Rs.380 are priced at Rs.20, the intrinsic value and time value of the options respectively are:
Intrinsic value = Rs.380 – Rs.370 = Rs.10